desigualdad de cauchy schwarz vectores ,15.6: Desigualdad de Cauchy,desigualdad de cauchy schwarz vectores,The Cauchy–Schwarz inequality (also called Cauchy–Bunyakovsky–Schwarz inequality) [1] [2] [3] [4] is an upper bound on the inner product between two vectors in an inner product space in . F# is an open-source, cross-platform, functional programming language for the .NET developer platform. Develop with free tools for Linux, macOS, and Windows.

Introduction
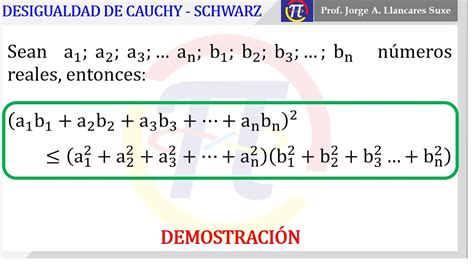
In mathematics, the Cauchy-Bunyakovsky-Schwarz inequality, often referred to as the Cauchy-Schwarz inequality or simply the Schwarz inequality, plays a fundamental role in various fields of mathematical analysis, including linear algebra, functional analysis, and probability theory. This inequality is pivotal because it provides essential bounds and relationships between vectors, helping to generalize the concept of orthogonality and magnitudes in inner product spaces. In this article, we will explore the Cauchy-Schwarz inequality specifically in the context of vectors, emphasizing its significance, proof, and applications.
Overview of the Cauchy-Schwarz Inequality
The Cauchy-Schwarz inequality, in its most common form, asserts that for any vectors \( \mathbf{u} \) and \( \mathbf{v} \) in an inner product space, the following inequality holds:
| \langle \mathbf{u}, \mathbf{v} \rangle | \leq \| \mathbf{u} \| \cdot \| \mathbf{v} \|
Here:
- \( \langle \mathbf{u}, \mathbf{v} \rangle \) denotes the inner product of the vectors \( \mathbf{u} \) and \( \mathbf{v} \),
- \( \| \mathbf{u} \| \) and \( \| \mathbf{v} \| \) represent the norms (or magnitudes) of the vectors \( \mathbf{u} \) and \( \mathbf{v} \), respectively.
This inequality establishes a relationship between the inner product of two vectors and the product of their magnitudes, and it holds for all vectors in an inner product space, including spaces of finite and infinite dimension.
Desigualdad de Cauchy: Historical Context and Variations
The Cauchy-Schwarz inequality was initially formulated by the French mathematician Augustin-Louis Cauchy in the 19th century, though its more complete form as we know it today was developed by several mathematicians, including Bunyakovsky and Schwarz. This inequality has seen applications not only in theoretical mathematics but also in diverse areas like physics, computer science, and statistics.
In Latin American countries and Spain, the inequality is often referred to as the "Desigualdad de Cauchy," a term which highlights Cauchy’s critical role in its formulation and popularization.
The Cauchy-Schwarz inequality is also closely related to the concepts of orthogonality and projection. It asserts that two vectors are orthogonal if and only if their inner product is zero, providing a fundamental tool for geometric interpretation in vector spaces.
Importance of Cauchy-Schwarz in Vector Spaces
In any inner product space, the Cauchy-Schwarz inequality helps determine the extent to which two vectors are related in terms of their directionality and magnitude. This is vital because it introduces the notion of cosine of the angle between two vectors. Specifically, the inequality can be rewritten in terms of the angle \( \theta \) between the vectors as:
| \langle \mathbf{u}, \mathbf{v} \rangle | = \| \mathbf{u} \| \| \mathbf{v} \| |\cos \theta|
This reformulation shows that the inner product is maximized when the vectors are parallel (i.e., when the angle \( \theta = 0 \)) and minimized when the vectors are orthogonal (i.e., when the angle \( \theta = 90^\circ \)).
Proof of the Cauchy-Schwarz Inequality
One of the most common and elegant proofs of the Cauchy-Schwarz inequality involves considering the following quadratic form. Let \( \mathbf{u} \) and \( \mathbf{v} \) be two vectors in an inner product space. Define a function \( f(t) \) as:
f(t) = \| \mathbf{u} - t \mathbf{v} \|^2
Expanding this quadratic expression:
f(t) = \langle \mathbf{u} - t \mathbf{v}, \mathbf{u} - t \mathbf{v} \rangle = \langle \mathbf{u}, \mathbf{u} \rangle - 2t \langle \mathbf{u}, \mathbf{v} \rangle + t^2 \langle \mathbf{v}, \mathbf{v} \rangle
This simplifies to:
desigualdad de cauchy schwarz vectores Browse through a vibrant retail mix, gastronomical wonders and innovative recreation choices at Pavilion Bukit Jalil. . The CHANEL BEAUTÉ Boutique is where you will meet the world of culture and creations of the House. Step inside a constantly evolving boutique and find a comprehensive range of CHANEL Fragrance, Makeup and Skincare.
desigualdad de cauchy schwarz vectores - 15.6: Desigualdad de Cauchy